Question

Calculate the z-test statistic for a hypothesis test in which the null hypothesis states that the population proportion, p, equals 0.14 if the following sample information is

present
n = 200
x= 31
(Round to two decimal places as needed.)
Z=

Answer 1

The z-test statistic for a hypothesis test in which the null hypothesis states that the population proportion, p, equals 0.14 if the following sample information is present (n = 200; x = 31) is z = .6114.

Explanation:

The z-test statistic formula is:

• 
  `\displaystyle z=\frac{\hat{p}-p}{\sqrt{(p(1-p))/(n) } }`

We are given x = 31 and n = 200, so we can solve for
`\hat{p}` : 31/200 = .155.

The population proportion,
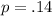`p=.14`, is given in the problem.

We can substitute the known values into the z test statistic formula:

• 
  `\displaystyle z = \frac{.155-.14}{\sqrt{(.14(.86))/(200) } }`
• 
  `\displaystyle z=\frac{.015}{\sqrt {.000602}}`
• 
  `z=.6114`

The z-test statistic for this problem is z = .6114.