Answer:
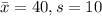
And from these values we can estimate the sample variance like this:
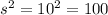
And we can also estimate the coeffcient of variation given by:

And replacing we got:
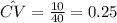
And this coefficient is useful in order to see the variability in terms of the mean for this case since is lower than 1 we can conclude that this variation around the mean is low.
Explanation:
For this case we have the following info given:
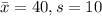
And from these values we can estimate the sample variance like this:
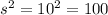
And we can also estimate the coeffcient of variation given by:

And replacing we got:
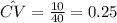
And this coefficient is useful in order to see the variability in terms of the mean for this case since is lower than 1 we can conclude that this variation around the mean is low.