Answer:
Explanation:
Let X denote the life span of a car battery and it follows and exponential distribution with average of 6 years.
Thus , the parameter of the exponential distribution is calculated as,
μ = 6
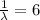
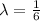
a) The required probability is
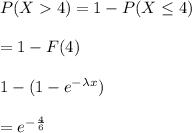
= 0.513
Hence, the probability that a randomly selected car battery will last more than four years is 0.513
b) The variance of the battery span is calculated as
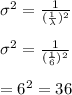
The 95% percentile
 (α = 5%) of the battery span is calculated
(α = 5%) of the battery span is calculated
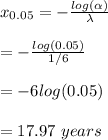
c)
Let
 denote the remaining life time of a car battery
denote the remaining life time of a car battery
i)the probability the battery will last an additional five years is calculated below

ii) The average time that the battery is expected to last is calculated
