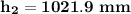Answer:
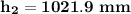
Step-by-step explanation:
Given that :
The initial volume of water
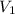 = 1000.00 mL = 1000000 mm³
= 1000.00 mL = 1000000 mm³
The initial temperature of the water
 = 10° C
= 10° C
The height of the water column h = 1000.00 mm
The final temperature of the water
 = 70° C
= 70° C
The coefficient of thermal expansion for the glass is ∝ =
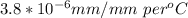
The objective is to determine the the depth of the water column
In order to do that we will need to determine the volume of the water.
We obtain the data for physical properties of water at standard sea level atmospheric from pressure tables; So:
At temperature
 the density of the water is
the density of the water is
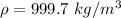
At temperature
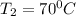 the density of the water is
the density of the water is
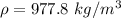
The mass of the water is
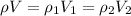
Thus; we can say
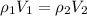 ;
;
⇒

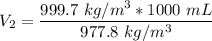
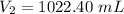
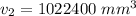
Thus, the volume of the water after heating to a required temperature of
 is 1022400 mm³
is 1022400 mm³
However; taking an integral look at this process; the volume of the water before heating can be deduced by the relation:
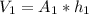
The area of the water before heating is:
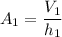
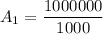
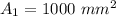
The area of the heated water is :
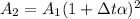
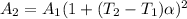
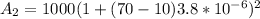
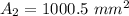
Finally, the depth of the heated hot water is:

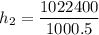
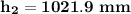
Hence the depth of the heated hot water is