Question:
A piston–cylinder device contains 0.85 kg of refrigerant- 134a at -10°C. The piston that is free to move has a mass of 12 kg and a diameter of 25 cm. The local atmospheric pressure is 88 kPa. Now, heat is transferred to refrigerant-134a until the temperature is 15°C. Determine (a) the final pressure, (b) the change in the volume of the cylinder, and (c) the change in the enthalpy of the refrigerant-134a.
Answer:
a) 90.4 kPa
b) 0.0205 m³
c) 17.4 kJ/kg
Step-by-step explanation:
Given:
Mass, m = 0.85 kg
a) The final pressure here is equal to the initial pressure. Let's use the formula:
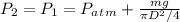

= 90398 Pa
≈ 90.4 KPa
Final pressure = 90.4 kPa
b) Change in volume of the cylinder:
To find the initial and final volume, let's use the values from the A-13 table for refrigerant-134a, at initial values of 90.4 kPa and -10°C and final values of 90.4 kPa and 15°C
v1 = 0.2302m³/kg
h1 = 247.76 kJ/kg
v2 = 0.2544 m³/kg
h2 = 268.2 kJ/kg
Change in volume is calculated as:
Δv = m(v2 - v1)
Δv = 0.85(0.2544 - 0.2302)
= 0.0205 m³
Change in volume = 0.0205 m³
c) Change in enthalpy
Let's use the formula:
Δh = m(h2 - h1)
= 0.85(268.2 - 247.76)
= 17.4 kJ/kg
Change in enthalpy = 17.4 kJ/kg