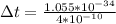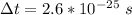Question

The Z0 boson, discovered in 1985, is the mediator of the weak nuclear force, and it typically decays very quickly. Its average rest energy is 91.19 GeV, but its short lifetime shows up as an intrinsic width of 2.5 GeV. what is the lifetime of this particle?

Answer 1

The lifetime of the particle is
`\Delta t = 2.6*10^(-25) \ s`

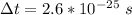
Step-by-step explanation:

From the question we are told that

The average rest energy is
`E = 91.19 \ GeV = 91.19GeV * (1.60 *10^(-10) J )/(1GeV) = 1.46 *10^(-8)J`

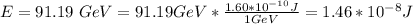
The intrinsic width is
`\Delta E =2.5eV = 2.5GeV * (1.60 *10^(-10)J )/(1GeV) = 4*10^(-10) J`

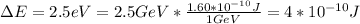
The lifetime is mathematically represented as

`\Delta t = (h)/(\Delta E)`

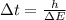
Where h is the Planck's constant with a value of
`1.055*10^(-34) \ J\cdot s`

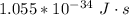
substituting values

`\Delta t = (1.055*10^(-34))/(4 *10^(-10))`

`\Delta t = 2.6*10^(-25) \ s`