Question

You are at a stop light in your car, stuck behind a red light. Just before the light is supposed to change, a fire engine comes zooming up towards you traveling at a horrendous 85.0 km/h. If the siren has a rated frequency 665 Hz, what frequency of the sound do you hear

Answer 1

The frequency of the sound you will hear is 713.85 Hz

Step-by-step explanation:

Given;

speed of your car,
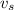`v_s` = 85.0 km/h

frequency of the siren, f = 665 Hz

Speed of sound in air, v = 345 m/s

The frequency of the sound you hear, can be calculated as;

`f' = f((v)/(v-v_s))`

Convert the speed of the car to m/s

`85 \ km/h =(85 \ km)/(h) ((1000\ m)/(1 \ km))((1 \ h)/(3600 \ s) ) = 23.61 \ m/s`

`f' = f((v)/(v-v_s) )\\\\f' = 665((345)/(345-23.61) )\\\\f' = 665 (1.07346)\\\\f' = 713.85 \ Hz`

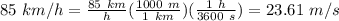
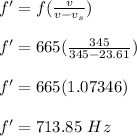
Therefore, the frequency of the sound you will hear is 713.85 Hz