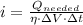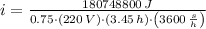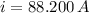Answer:
a)
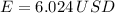 , For m kilograms, it is 4184m J., 3600000 joules, b)
, For m kilograms, it is 4184m J., 3600000 joules, b)
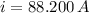
Step-by-step explanation:
a) The amount of heat needed to warm water is given by the following expression:

Where:
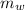 - Mass of water, measured in kilograms.
- Mass of water, measured in kilograms.
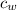 - Specific heat of water, measured in
- Specific heat of water, measured in
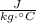 .
.
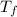 ,
,
 - Initial and final temperatures, measured in
- Initial and final temperatures, measured in
 .
.
Then,

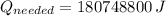
The energy needed in kilowatt-hours is:

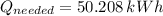
The electric energy required to heat up the water is:
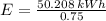
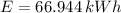
Lastly, the cost of heating a hot tub is: (USD - US dollars)
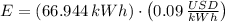
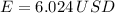
The heat needed to raise the temperature a degree of a kilogram of water is 4184 J. For m kilograms, it is 4184m J. Besides, a kilowatt-hour is equal to 3600000 joules.
b) The current required for the electric heater is: