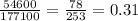Question

A university warehouse has received a shipment of 25 printers, of which 10 are laser printers and 15 are inkjet models. If 6 of these 25 are selected at random to be checked by a particular technician, what is the probability that exactly 3 of those selected are laser printers (so that the other 3 are inkjets)

Answer 1

The probability is 0.31

Explanation:

To find the probability, we will consider the following approach. Given a particular outcome, and considering that each outcome is equally likely, we can calculate the probability by simply counting the number of ways we get the desired outcome and divide it by the total number of outcomes.

In this case, the event of interest is choosing 3 laser printers and 3 inkjets. At first, we have a total of 25 printers and we will be choosing 6 printers at random. The total number of ways in which we can choose 6 elements out of 25 is
`\binom{25}{6}`, where
`\binom{n}{k} = (n!)/((n-k)!k!)`. We have that
`\binom{25}{6} = 177100`

Now, we will calculate the number of ways to which we obtain the desired event. We will be choosing 3 laser printers and 3 inkjets. So the total number of ways this can happen is the multiplication of the number of ways we can choose 3 printers out of 10 (for the laser printers) times the number of ways of choosing 3 printers out of 15 (for the inkjets). So, in this case, the event can be obtained in
`\binom{10}{3}\cdot \binom{15}{3} = 54600`

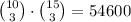
So the probability of having 3 laser printers and 3 inkjets is given by

`(54600)/(177100) = (78)/(253) = 0.31`