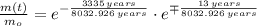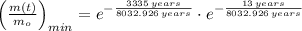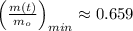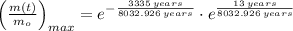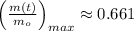Question

adiocarbon dating of blackened grains from the site of ancient Jericho provides a date of 1315 BC ± 13 years for the fall of the city. What is the relative amount of 14C in the old grain vs the new grain in 2007 AD? (A0 = original radioactivity; At = radioactivity in 2007 AD).

Answer 1

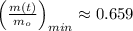`\left((m(t))/(m_(o)) \right)_(min) \approx 0.659` and
`\left((m(t))/(m_(o)) \right)_(max) \approx 0.661`

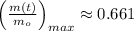
Explanation:

The equation of the isotope decay is:

`(m(t))/(m_(o)) = e^{-(t)/(\tau) }`

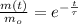
14-Carbon has a half-life of 5568 years, the time constant of the isotope is:

`\tau = (5568\,years)/(\ln 2)`

`\tau \approx 8032.926\,years`

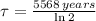
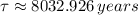
The decay time is:

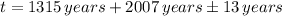`t = 1315\,years + 2007\,years \pm 13\,years` (There is no a year 0 in chronology).

`t = 3335 \pm 13\,years`

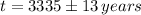
Lastly, the relative amount is estimated by direct substitution:

`(m(t))/(m_(o)) = e^{-(3335\,years)/(8032.926\,years) }\cdot e^{\mp(13\,years)/(8032.926\,years) }`

`\left((m(t))/(m_(o)) \right)_(min) = e^{-(3335\,years)/(8032.926\,years) }\cdot e^{-(13\,years)/(8032.926\,years) }`

`\left((m(t))/(m_(o)) \right)_(min) \approx 0.659`

`\left((m(t))/(m_(o)) \right)_(max) = e^{-(3335\,years)/(8032.926\,years) }\cdot e^{(13\,years)/(8032.926\,years) }`

`\left((m(t))/(m_(o)) \right)_(max) \approx 0.661`