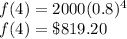Answer:
(A)Decay
(b)0.8
(c)First Term
(d)
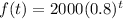
(e)$819.20
Explanation:
The exponential function for modelling growth or decay is given as:
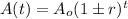 ,
,
Where:
Plus indicates growth and minus indicates decay.
For a powerful computer that was purchased for $2000, but loses 20% of its value each year.
(a)Since it loses value, it is a decay.
(b)Multiplier
Its value decays by 20%.
Therefore, our multiplier(1-r) =(1-20&)=1-0.2
Multiplier =0.8
(c)$2000 is our First term (or Initial Value
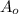 )
)
(d)The function for this problem is therefore:
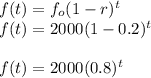
(e)Since we require the worth of the computer after 4 years,
t=4 years