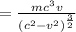Complete Question
The complete question is shown on the first uploaded image
Answer:
a
![(\delta E)/(\delta m)= c^2 [\frac{1}{\sqrt{1 - (v^2)/(c^2) } } -1 ]](https://img.qammunity.org/2021/formulas/mathematics/college/mmwt2mw0tj4yd13la3pcgcpma2wor04rc9.png)
b
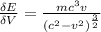
Explanation:
From the question we are given
![E = mc^2 [\frac{1}{\sqrt{1 - (v^2)/(c^2) } }- 1 ]](https://img.qammunity.org/2021/formulas/mathematics/college/865xsc93f6qmyrksav8lel61v8kp6ukzdd.png)
So we are asked to find

Now this is mathematically evaluated as
![(\delta E)/(\delta m) = (\delta )/(\delta m) [mc^2 ( \frac{1}{\sqrt{1 - (v^2)/(c^2) } } -1 )]](https://img.qammunity.org/2021/formulas/mathematics/college/anf4xbpdaim12ptbpt37qhpxz7rpo31xi8.png)
![= c^2 [\frac{1}{\sqrt{1 - (v^2)/(c^2 ) } } -1 ] (\delta m)/(\delta m)](https://img.qammunity.org/2021/formulas/mathematics/college/ptcrypzva6qss2ifcj7xi95fv192fjvda7.png)
![= c^2 [\frac{1}{\sqrt{1 - (v^2)/(c^2) } } -1 ]](https://img.qammunity.org/2021/formulas/mathematics/college/fi1xn63qlan3dgbmhxlssvd40froxrlhbp.png)
Also we are asked to find

Now this is mathematically evaluated as
![(\delta E)/(\delta V) = (\delta )/(\delta v ) [mc^2 ( \frac{1}{\sqrt{1 - (v^2)/(c^2) } } - 1 )]](https://img.qammunity.org/2021/formulas/mathematics/college/mrb248yariv1lljxqtyhrzqc3829xam9f7.png)
![(\delta E)/(\delta V) = mc^2 [(\delta )/(\delta v) ((c)/(√(c^2 -v^2) ) - 1 )]](https://img.qammunity.org/2021/formulas/mathematics/college/wabds7h31tit2im4nypb1vy2p897v25oz6.png)
![= mc^2 [c* [(\delta )/(\delta v) (c^2 - v^2 )^{-(1)/(2) }] - 0]](https://img.qammunity.org/2021/formulas/mathematics/college/vfaqk7xbns50066h6ejy5m0aflsd4vn4m4.png)
![= mc^3 [- (1)/(2) (c^2 - v^2 )^{-(3)/(2) } * (-2v)]](https://img.qammunity.org/2021/formulas/mathematics/college/jefw77ser12tacqq26u4sozxqz0h1nm4gd.png)