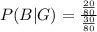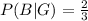Complete Question
Calculating conditional probability
The usher at a wedding asked each of the 80 guests whether they were a friend of the bride or of the groom.
Here are the results:
Bride :29
Groom :30
BOTH : 20
Given that a randomly selected guest is a friend of the groom, find the probability they are a friend of the bride.
P (bride | groom)
Answer:
The probability is
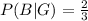
Explanation:
The sample size is

The friend of the groom are
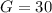
The friend of the groom are
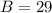
The friend of both bride and groom are
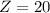
The probability that a guest is a friend of the bride is mathematically represented as
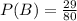
The probability that a guest is a friend of the groom is mathematically represented as
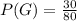
The probability that a guest is both a friend of the bride and a friend of the groom is mathematically represented as
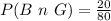
Now
 is mathematically represented as
is mathematically represented as
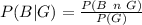
Substituting values