Answer:
Step-by-step explanation:
1.
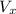 =
=
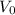 * cos
* cos
 ⇒ 16*cos32 ≈ 13.6 m/s (13.56)
⇒ 16*cos32 ≈ 13.6 m/s (13.56)
2.
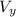 =
=
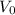 * sin
* sin
 ⇒ 16* sin32 ≈ 9.4 m/s
⇒ 16* sin32 ≈ 9.4 m/s
3.
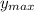 =
=
 =
=
 (the g (gravity) depends on the country but i'll take the average g which is 9.2m/s^2)
(the g (gravity) depends on the country but i'll take the average g which is 9.2m/s^2)
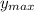 ≈ 3.6677+1.5 ≈ 5.2m
≈ 3.6677+1.5 ≈ 5.2m
4.
 =
=
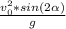 =
=
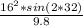 ≈ 23.5m (23.47)
≈ 23.5m (23.47)
5. -
answer 4 could be wrong, not certain about that one and i don't know 5