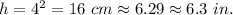Answer:6.3 in.
Explanation:
Given
Radius of sphere
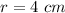
When ball is immersed in beaker, it causes the water to flowout of beaker which is collected in the conical vessel
Such that amount of water equivalent to sphere volume is collected in it.
volume of ball
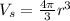
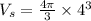
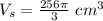
Now volume of water collected is in shape of cone then volume of cone

and
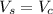
![(256\pi )/(3)=(\pi )/(3)(4)^2* h\ quad [\text{h=height of cone formed}]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/f4w24b33osw36qkpwhcp9dhs4kmch1bmni.png)