Question

an amount was invested at r% per quarter. what value of r will ensure that accumulated amount at the end of one year is 1.5 times more than amount invested

Answer 1

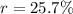`r=25.7\%` will ensure that accumulated amount at the end of one year is 1.5 times more than amount invested

Explanation:

Given: An amount was invested at r% per quarter.

To find: value of r such that accumulated amount at the end of one year is 1.5 times more than amount invested

Solution:

Let P denotes amount invested and n denotes time

As an amount (A) was invested at r% per quarter,

`A=P\left ( 1+(r)/(400) \right )^(4n)`

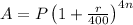
According to question, accumulated amount at the end of one year is 1.5 times more than amount invested.

So,

`A=1.5P+P=2.5P\\A=2.5P\\P\left ( 1+(r)/(400) \right )^(4n)=2.5P`

Put n = 1

`P\left ( 1+(r)/(400) \right )^(4)=2.5P\\\left ( 1+(r)/(400) \right )^(4)=2.5\\1+(r)/(400) =(2.5)^{(1)/(4)}\\(r)/(100)=(2.5)^{(1)/(4)}-1\\r=100\left [ (2.5)^{(1)/(4)}-1 \right ]\\=25.7\%`