Answer:
a) t = 27.00 h
b) B = 6.84 MeV/nucleon
Step-by-step explanation:
a) The time can be calculated using the following equation:
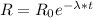
Where:
R: is the radiation measured at time t
R₀: is the initial radiation
λ: is the decay constant
t: is the time
The decay constant can be calculated as follows:
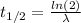
Where:
t(1/2): is the half life = 4.5 h

We have that the radiation measured is 64 times the maximum permissible level, thus R₀ = 64R:
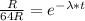
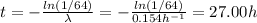
b) The binding energy (B) can be calculated using the following equation:

Where:
Z: is the number of protons = 2 (for
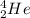 )
)
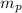 : is the proton mass = 1.00730 u
: is the proton mass = 1.00730 u
N: is the number of neutrons = 2 (for
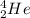 )
)
 : is the neutron mass = 1.00869 u
: is the neutron mass = 1.00869 u
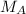 : is the mass of the He atom = 4.002602 u
: is the mass of the He atom = 4.002602 u
A = N + Z = 2 + 2 = 4
The binding energy of
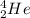 is:
is:
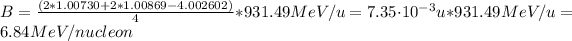
Hence, the binding energy per nucleon is 6.84 MeV.
I hope it helps you!