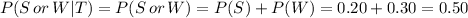Question

A sports car manufacturer paints its cars silver, white, black, and red in the following proportions: ?

Color: Silver White Black Red
Proportion: .2 .3 .1 .4
We know that 40% of these cars are manufactured with tan leather upholstery while the remaining 60% are manufactured with gray leather.
A. Assuming that the choice of exterior color and leather color are independent, what is the probability that a randomly selected sports car from this manufacturer will be white with gray upholstery?
B. Assuming that we know the car has tan upholstery, what is the probability that the car is either silver or white?

Answer 1

A. The probability that a randomly selected sports car from this manufacturer will be white with gray upholstery is P=0.12.

B. Assuming that we know the car has tan upholstery, the probability that the car is either silver or white is P=0.50.

Explanation:

We first start by stating that the events "exterior color" and "leather color" are independent, so the probability of the outcomes of each event is not affected by the outcomes of the other event.

A. The probability of having a car that is white (W) with gray upholstery (G) is equal to the probability of having a car that is white multiplied by the probability of having a car with gray leather upholstery. Mathematically, this is:

`P(\text{W\&G})=P(W)\cdot P(G)=0.3\cdot 0.4=0.12`

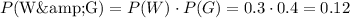
B. As the events are independent, the probability of having a silver or white car, given that the car has tan upholstery, is the same as the probabiltiy of having a silver or white car:

`P(S\,or\,W | T)=P(S\,or\,W)=P(S)+P(W)=0.20+0.30=0.50`