Question

A machine has four components, A, B, C, and D, set up in such a manner that all four parts must work for the machine to work properly. Assume the probability of one part working does not depend on the functionality of any of the other parts. Also assume that the probabilities of the individual parts working are P(A) = P(B) = 0.93, P(C) = 0.95, and P(D) = 0.92. Find the probability that the machine works properly.A) 0.8128B) 0.2441C) 0.8217D) 0.7559

Answer 1

D) 0.7559

Explanation:

Independent events:

If two events, A and B are independent:

`P(A \cap B) = P(A)*P(B)`

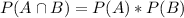
In this question:

Four independent events, A, B, C and D.

So

`P(A \cap B \cap C \cap D) = P(A)*P(B)*P(C)*P(D)`

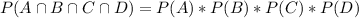
Find the probability that the machine works properly.

This is the probability that all components work properly.

`P(A \cap B \cap C \cap D) = P(A)*P(B)*P(C)*P(D) = 0.93*0.93*0.95*0.92 = 0.7559`

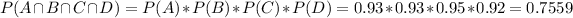
So the correct answer is:

D) 0.7559