Question

10.1 Populations and Samples

1. A company uses a computer to identify their 600 most loyal customers
from its database and then surveys those customers to find out how
10.2 Making Inferences from a Random Sample
2. A university has 30,330 students. In a random sample of 270 students,
18 speak three or more languages. Predict the number of students
at the university who speak three or more languages.
10.3 Generating Random Samples
A store receives a shipment of 5,000 MP3 players. In a previous shipment
numbers 1 through 300 represent defective MP3 players, and the numbers
numbers to simulate a random sample of this shipment. The clerk lets the
301 through 5,000 represent working MP3 players. The results are given.
13 2,195 3,873 525 900 167 1,094 1,472 7095,000
they like their service. Identify the population and determine whether
of 5,000 MP3 players, 300 were defective. A store clerk generates random
3. Based on the sample, how many of the MP3 players might the clerk
4. Can the manufacturer assume the prediction is valid? Explain.
5. How can you use random samples to solve real-world problems?
the sample is random or biased,
predict would be defective?
ESSENTIAL QUESTION

Answer 1

I think you can multiply how many are defective in a sample by how much more stuff there is

Answer 2

I'm glad you asked!I couldn't fully understand it so I made it the best of my ability.

Explanation:

1.(Please,I cannot understand it .I think it is incomplete.)

2.18 out of 270 students speak 3 languages or more.So
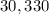`30,330` x
`(18)/(270)` =
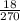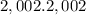`2,002.2,002` students.

3.We would do 5,000 x
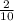`(2)/(10)` = 1,000.

4.No, the manufacturer cannot assume the prediction is valid.

The sample size of 10 is too small compared to the size of the shipment.

5.You can use random samples by using them for graphs which numbers are too big.