Question

A horizontal line has points J, K, L. A line extends from point K up and to the right to point M. Angle J K M is (10 y + 6) degrees and angle M K L is (8 y minus 6) degrees

What is the value of y?
y =

Find the following angle measures.
mAngleJKM = °
mAngleMKL = °

Answer 1

The found values are:

y = 10

<JKM = 106°

<MKL = 74°

Explanation:

(See the diagram attached)

As JKL is a straight horizontal line, angle measure from JK to KL is 180°.

We can see in the diagram that line KM is dividing this angle of 180° into 2 unequal parts.

Which means that the sum of <JKM and <MKL is equals to 180°.

Mathematically, it can be written as:

`<JKM+<MKL=180^\circ\\\\`

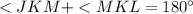
Substitute the values of both angle to solve the equation:

`(10y+6)+(8y-6) = 180\\18y=180\\y=(180)/(18)\\y=10`

Put in the formulas of both angle to find their values:

<JKM = 10y + 6

<JKM = 10(10)+6

<JKM = 106°

<MKL = 8y - 6

<MKL = 8(10)-6

<MKL = 74°