Answer:
A. we will see that the notion
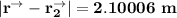 which denotes Stephen Curry illustrates that Stephen Curry minigolf ball shot is closer
which denotes Stephen Curry illustrates that Stephen Curry minigolf ball shot is closer
B. Lebron James hits at an angle of 17.48° North -East.
The direction of Stephen is = 20° due to East of North
Step-by-step explanation:
Let
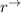 represent the position vector of the hole;
represent the position vector of the hole;
Also; using the origin as starting point. Let the east direction be along the positive x axis and the North direction be + y axis
Thus:
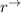 =
=

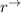 =
=
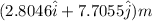
Let
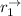 be the position vector for Lebron James's first shot
be the position vector for Lebron James's first shot
So;
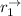 =
=
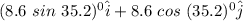
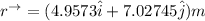
Let
 be the position vector for Stephen Curry's shot
be the position vector for Stephen Curry's shot

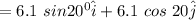
 =
=
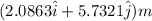
However;
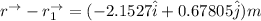
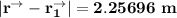
Also;
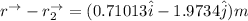
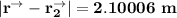
Thus; from above ; we will see that the notion
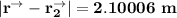 which denotes Stephen Curry illustrates that Stephen Curry minigolf ball shot is closer
which denotes Stephen Curry illustrates that Stephen Curry minigolf ball shot is closer
B .
For Lebron James ;
The angle can be determine using the trigonometric function:
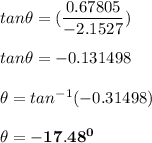
Thus Lebron James hits at an angle of 17.48° North -East.
For Stephen Curry;
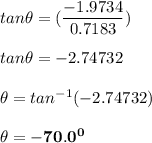
The direction of Stephen is = 90° - 70° = 20° due to East of North