Question

The sum of an infinite geometric sequence is seven times the value of its first term.

a) Find the common ratio of the sequence.
b) Find the least number of terms of the sequence that must be added in order for the sum to exceed half the value of
the infinite sum.

Answer 1

a). r =
`(6)/(7)`

b). At least 5 terms should be added.

Explanation:

Formula representing sum of infinite geometric sequence is,

`S_(\inf)=(a)/(1-r)`

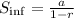
Where a = first term of the sequence

r = common ratio

a). If the sum is seven times the value of its first term.

`7a=(a)/(1-r)`

`7=(1)/(1-r)`

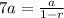
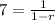
7(1 - r) = 1

7 - 7r = 1

7r = 7 - 1

7r = 6

r =
`(6)/(7)`

b). Since sum of n terms of the geometric sequence is given by,

`S_(n)=(a(1-r^(n)))/(1-r)`

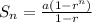
If the sum of n terms of this sequence is more than half the value of the infinite sum.

`(a[1-((6)/(7))^(n)])/(1-(6)/(7))` >
`(7a)/(2)`

`(1-((6)/(7))^(n))/(1-(6)/(7))> (7)/(2)`

`(1-((6)/(7))^(n))/((1)/(7))> (7)/(2)`

`1-((6)/(7))^(n)> (7)/(2)* (1)/(7)`

`1-((6)/(7))^(n)> (1)/(2)`

`-((6)/(7))^(n)> -(1)/(2)`

`((6)/(7))^(n)< (1)/(2)`

`(0.85714)^(n)< (0.5)`

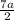
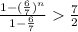
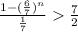
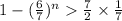
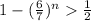
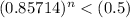
n[log(0.85714)] < log(0.5)

-n(0.06695) < -0.30102

n >
`(0.30102)/(0.06695)`

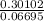
n > 4.496

n > 4.5

Therefore, at least 5 terms of the sequence should be added.