Question

Which is the ordered pair for the point on the x-axis that is on the line parallel to the given line and through the given point (-6,10)

Answer 1

Explanation:

The answer was (6,0) I just got it right on my assignment

Answer 2

Option (1). (6, 0)

Explanation:

The given question is incomplete; here is the complete question.

Which is the ordered pair for the point on the x-axis that is on the line parallel to the given line and through the given point (–6, 10)?

(6, 0)

(0, 6)

(−5, 0)

(0, −5)

Line in orange color is passing through two points (-8, 6) and (4, -4)

Slope of this line =
`(\triangle y)/(\triangle x)`

=
`(6+4)/(-8-4)`

=
`-(10)/(12)`

=
`-(5)/(6)`

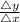
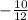
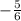
Other line parallel to this line will have the same slope 'm' =
`-(5)/(6)`

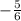
Parallel line passes through a point (-6, 10).

Let the other point through which the parallel line passes is (a, b)

Now,
`-(5)/(6)` =
`(10-b)/(-6-a)`

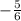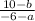
-5(-a - 6) = 6(10 - b)

5a + 30 = 60 - 6b

5a = -6b + 60 - 30

5a = -6b + 30

a =
`-(6)/(5)b+6`

By satisfying with all the options we find only (6, 0) satisfy this equation.

6 =
`-(6)/(5)(0)+6`

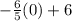
6 = 6

Therefore, (6, 0) is the other point lying on the parallel line.

Option (1) will be the answer.