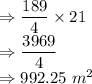Question

The diagram shown represents a garden. The scale is 2/3 centimeters for every 3 1/2 meters. Each square in the drawing measures 1cm by 1cm. Find the actual length, width and area of the garden based upon the given drawing.

Answer 1

Length =
`(189)/(4)\ m`

Width = 21 m

Area of garden = 992.25
`m^(2)`

Explanation:

Side of square = 1 cm

As per the given question figure, we can see that there are 11 square across the length of rectangular garden.

But the garden includes only 9 squares, the corner squares are not in the garden.

So, length of garden = 9
`*` 1 = 9 cm

Using the scaling given in the question,

`(2)/(3)\ cm = 3(1)/(2)\ m\\`
`\Rightarrow (2)/(3)\ cm = (7)/(2)\ m`

`1 cm = ((7)/(2))/((2)/(3)) \ m \Rightarrow (21)/(4)\ m`

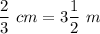
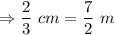
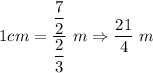
So, length of garden =
`(21)/(4) * 9 \Rightarrow (189)/(4)\ m`

-------------

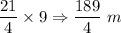
And, similarly width contains 4 squares, (Corner squares are excluded)

Width of garden =
`(21)/(4) * 4 \Rightarrow 21\ m`

-------------

Area of garden = Length
`*` Width

`\Rightarrow (189)/(4) * 21\\\Rightarrow (3969)/(4)\\\Rightarrow 992.25\ m^(2)`