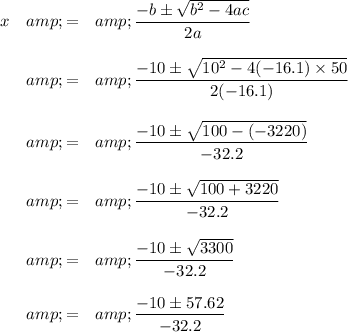Answer:
2.1 s
Explanation:
1. Write the equation
The formula for vertical displacement is
y = h + v₀t - ½gt²
h = 50 ft
v₀ = 10 ft/s
g = 32.2 ft/s²
Insert the values
y = 50 + 10t - ½ × 32.2t²
y = 50 + 10t - 16.1t²
The graph below shows the height as a function of time.
2. Solve the equation
a = -16.1; b = 10; c = 50