Question

A frequency distribution for the class level of students in an introductory statistics course is shown. Two students are randomly selected without replacement. Complete parts​ (a) through​ (d).

Class
Frequency

Freshman
6
Sophomore
16
Junior
12
Senior
7
a. Determine the probability that the first student obtained is a junior and the second a senior.

Answer 1

Answer:
`(21)/(410)`.

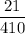Explanation:

Formula : Probability =
`\frac{\text{favorable outcomes}}{\text{Total outcomes}}`

From the given frequency distribution for the class level of students in an introductory statistics course, we have

Number of Junior= 12

Number of Senior = 7

Total students = 6+16+12+7 = 41

Probability that the first student obtained is a junior =
`(12)/(41)`

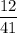
Probability that the the second student obtained is a senior.
`=(7)/(41-1)=(7)/(40)`

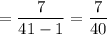
Then, the probability that the first student obtained is a junior and the second a senior would be
`(12)/(41)*(7)/(40)=(21)/(410)`

Hence, the required probability is
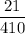`(21)/(410)`.