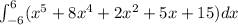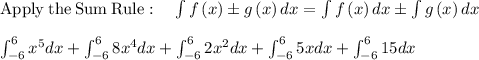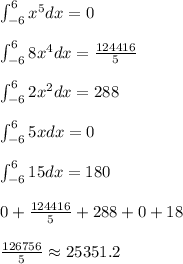Question

Use the fundamental theorem of calculus to find the area of the region between the graph of the function x^5 + 8x^4 + 2x^2 + 5x + 15 and the x-axis on the interval [-6,6]. Round off your answer to the nearest integer.

A) 25,351 units^2
B) 149,473 units^2
C) 3,758 units^2
D) 2,362 units^2

Answer 1

To find the area of the region between a curve and the x-axis on a certain interval, we need to calculate the definite integral of the function defining the curve over that interval. The fundamental theorem of calculus allows us to do exactly that.

Here we are asked to find the area between the curve defined by f(x) = x^5 + 8x^4 + 2x^2 + 5x + 15 and the x-axis over the interval from x = -6 to x = 6.

The definite integral of f(x) from a to b is given by the integral sign with the lower limit a and the upper limit b, like this:

∫[a to b] f(x) dx

In our case, to find the area between the curve and the x-axis, we will calculate the following:

∫[-6 to 6] (x^5 + 8x^4 + 2x^2 + 5x + 15) dx

After integrating the function term by term with respect to x, we would have a new function F(x), which is known as an antiderivative of f(x). Then, by the fundamental theorem of calculus, we evaluate F(x) at the upper limit (x = 6) and subtract its evaluation at the lower limit (x = -6) to get the exact area.

The antiderivative F(x), without the constant of integration since it cancels out in the subtraction, would look something like:

F(x) = (1/6)x^6 + (8/5)x^5 + (2/3)x^3 + (5/2)x^2 + 15x

Let's denote F(x) evaluated at 6 as F(6) and at -6 as F(-6). The area A is then A = F(6) - F(-6).

After performing these calculations and evaluating the antiderivative at x=6 and x=-6, we have the definite integral value which represents the exact area between the curve f(x) and the x-axis over the interval [-6, 6].

Rounding this value off to the nearest integer gives us the result for the area as 25,351 square units.

Hence, the correct answer to the question is:

A) 25,351 units^2

Answer 2

The area of the region is 25,351
`units^2`.

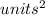Explanation:

The Fundamental Theorem of Calculus: if
`f` is a continuous function on
`[a,b]`, then

`\int_(a)^(b) f(x)dx = F(b) - F(a) = F(x) | {_a^b}`

where
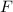`F` is an antiderivative of
`f`.

A function
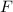`F` is an antiderivative of the function
`f` if

`F^(')(x)=f(x)`

The theorem relates differential and integral calculus, and tells us how we can find the area under a curve using antidifferentiation.

To find the area of the region between the graph of the function
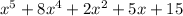`x^5 + 8x^4 + 2x^2 + 5x + 15` and the x-axis on the interval [-6, 6] you must:

Apply the Fundamental Theorem of Calculus

`\int _(-6)^6(x^5+8x^4+2x^2+5x+15)dx`

`\mathrm{Apply\:the\:Sum\:Rule}:\quad \int f\left(x\right)\pm g\left(x\right)dx=\int f\left(x\right)dx\pm \int g\left(x\right)dx\\\\\int _(-6)^6x^5dx+\int _(-6)^68x^4dx+\int _(-6)^62x^2dx+\int _(-6)^65xdx+\int _(-6)^615dx`

`\int _(-6)^6x^5dx=0\\\\\int _(-6)^68x^4dx=(124416)/(5)\\\\\int _(-6)^62x^2dx=288\\\\\int _(-6)^65xdx=0\\\\\int _(-6)^615dx=180\\\\0+(124416)/(5)+288+0+18\\\\(126756)/(5)\approx 25351.2`