Question

For a certain good we have LaTeX: q=f\left(p\right)=200e^{-0.4p}q = f ( p ) = 200 e − 0.4 p. a) Find the elasticity of demand at price p = $50. b) At p = $50, is the demand elastic, inelastic, or does it have unit elasticity? Explain what this means for this product. c) Find the elasticity of demand at price p = $20. d) At p = $20, is the demand elastic, inelastic, o

Answer 1

a) 20

b) elastic

c) 8

d) elastic

Step-by-step explanation:

Given that q = f ( p ) =
`200e^(-0.4p)`

`(dq)/(dp) = -80e^(-0.4p)`

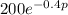
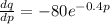
a) The elasticity of demand is given as:

Elasticity of demand =
`(dq)/(dp)*(p)/(q)`

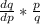
At p =$50,
`(dq)/(dp) = -80e^(-0.4p)=-80e^(-0.4*50)=-1.65*10^(-7)`

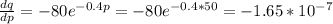
q = f ( p ) =
`200e^(-0.4p)` =
`200e^(-0.4*50)=-4.12*10^(-7)`

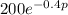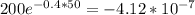
Elasticity of demand =
`(dq)/(dp)*(p)/(q)` =
`-1.65*10^(-7)*(50)/(-4.12*10^(-7))=20`

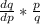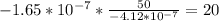
b) At p = $50, it is elastic Since Elasticity of demand is greater than 1 it is elastic. That is the price have a big effect on the quantity

c) The elasticity of demand is given as:

Elasticity of demand =
`(dq)/(dp)*(p)/(q)`

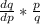
At p =$20,
`(dq)/(dp) = -80e^(-0.4p)=-80e^(-0.4*20)=-0.027}`

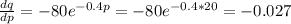
q = f ( p ) =
`200e^(-0.4p)` =
`200e^(-0.4*20)=-0.067`

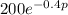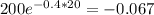
Elasticity of demand =
`(dq)/(dp)*(p)/(q)` =
`-0.027*(20)/(-0.067)=8.0`

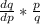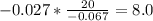
d) At p = $20, it is elastic Since Elasticity of demand is greater than 1 it is elastic