Question

Determine whether the equation is exact. If it​ is, then solve it. e Superscript t Baseline (7 y minus 3 t )dt plus (2 plus 7 e Superscript t Baseline )dy equals 0et(7y−3t)dy2+7et dy=0 Select the correct choice below​ and, if​ necessary, fill in the answer box to complete your choice.

Answer 1

`F(t,y)=(2+7e^t)y+3(1-t)e^t +C`

Explanation:

You have the following differential equation:

`e^t(7y-3t)dt+(2+7e^t)dy=0`

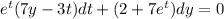
This equation can be written as:

`Mdt+Ndy=0`

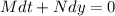
where

`M=e^t(7y-3t)\\\\N=(2+7e^t)`

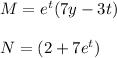
If the differential equation is exact, it is necessary the following:

`(\partial M)/(\partial y)=(\partial N)/(\partial t)`

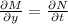
Then, you evaluate the partial derivatives:

`(\partial M)/(\partial y)=(\partial)/(\partial t)e^t(7y-3t)\\\\(\partial M)/(\partial t)=7e^t\\\\(\partial N)/(\partial t)=(\partial)/(\partial t)(2+7e^t)\\\\(\partial N)/(\partial t)=7e^t\\\\(\partial M)/(\partial t) = (\partial N)/(\partial t)`

The partial derivatives are equal, then, the differential equation is exact.

In order to obtain the solution of the equation you first integrate M or N:

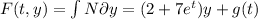`F(t,y)=\int N \partial y = (2 +7e^t)y+g(t)` (1)

Next, you derive the last equation respect to t:

`(\partial F(t,y))/(\partial t)=7ye^t+g'(t)`

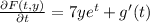
however, the last derivative must be equal to M. From there you can calculate g(t):

`(\partial F(t,y))/(\partial t)=M=(7y-3t)e^t=7ye^t+g'(t)\\\\g'(t)=-3te^t\\\\g(t)=-3\int te^tdt=-3[te^t-\int e^tdt]=-3[te^t-e^t]`

Hence, by replacing g(t) in the expression (1) for F(t,y) you obtain:

`F(t,y)=(2+7e^t)y+3(1-t)e^t +C`

where C is the constant of integration