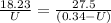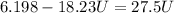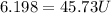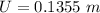Answer:
The y-value of the line in the xy-plane where the total magnetic field is zero
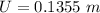
Step-by-step explanation:
From the question we are told that
The distance of wire one from two along the y-axis is y = 0.340 m
The current on the first wire is
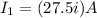
The force per unit length on each wire is
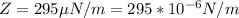
Generally the force per unit length is mathematically represented as
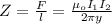
=>

Where
 is the permeability of free space with a constant value of
is the permeability of free space with a constant value of
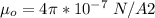
substituting values
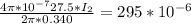
=>
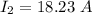
Let U denote the line in the xy-plane where the total magnetic field is zero
So
So the force per unit length of wire 2 from line U is equal to the force per unit length of wire 1 from line (y - U)
So
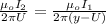
substituting values