Question

A football team consists of 18 freshmen and 18 sophomores, 15 juniors, and 12 seniors. Four players are selected at random to serve as captains. Find the probability that at least 1 of the students is a senior.

Answer 1

58.05% probability that at least 1 of the students is a senior.

Explanation:

A probability is the number of desired outcomes divided by the number of total outcomes.

The order in which the captains are chosen is not important. So we use the combinations formula to solve this question.

Combinations formula:

A probability is the number of desired outcomes divided by the number of total outcomes.

In this question:

Either no seniors are captain, or at least one is. The sum of these probabilities is 100%. The easier way to solve this question is finding the probability of no seniors being captain, and subtratcing 100 from this. So

Probability of no seniors being captain:

Desired outcomes:

Four captains, from a set of 18 + 18 + 15 = 51. So

`D = C_(51,4) = (51!)/(4!(51-4)!) = 249900`

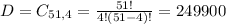
Total outcomes:

Four captains from a set of 18 + 18 + 15 + 12 = 63. So

`T = C_(63,4) = (63!)/(4!(63-4)!) = 595665`

Probability:

`p = (D)/(T) = (249900)/(595665) = 0.4195`

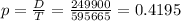
41.95% probability of no seniors being captains.

Find the probability that at least 1 of the students is a senior.

p + 41.95 = 100

p = 58.05

58.05% probability that at least 1 of the students is a senior.