Question

Suppose you have a sample of 27 Syrian hamsters who were exposed to high levels of the hormone progesterone when they were pups, and who have an average gestation length of 17.4 days and a sample variance of 33.7 days. You want to test the hypothesis that Syrian hamsters who were exposed to high levels of the hormone progesterone when they were pups have a different gestation length than all Syrian hamsters.

Required:
Calculate the t- statistics.

Answer 1

Test statistic t=1.2531.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that Syrian hamsters who were exposed to high levels of the hormone progesterone when they were pups have a different gestation length than all Syrian hamsters (P-value=0.11).

Explanation:

The question is incomplete: We need the gestation length of the population to perform the hypothesis test. We assume it to be 16 days (μ=16).

This is a hypothesis test for the population mean.

The claim is that Syrian hamsters who were exposed to high levels of the hormone progesterone when they were pups have a different gestation length than all Syrian hamsters.

Then, the null and alternative hypothesis are:

`H_0: \mu=16\\\\H_a:\mu> 16`

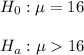
The significance level is 0.05.

The sample has a size n=27.

The sample mean is M=17.4.

The sample variance is s^2=33.7 days^2.

As the standard deviation of the population is not known, we estimate it with the sample standard deviation, that has a value of s=33.7^(0.5)=5.81.

The estimated standard error of the mean is computed using the formula:

`s_M=(s)/(√(n))=(5.81)/(√(27))=1.1172`

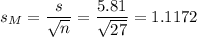
Then, we can calculate the t-statistic as:

`t=(M-\mu)/(s/√(n))=(17.4-16)/(1.1172)=(1.4)/(1.1172)=1.2531`

The degrees of freedom for this sample size are:

`df=n-1=27-1=26`

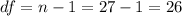
This test is a right-tailed test, with 26 degrees of freedom and t=1.2531, so the P-value for this test is calculated as (using a t-table):

`P-value=P(t>1.2531)=0.11`

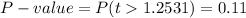
As the P-value (0.11) is bigger than the significance level (0.05), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that Syrian hamsters who were exposed to high levels of the hormone progesterone when they were pups have a different gestation length than all Syrian hamsters.