Answer:
1:4
Step-by-step explanation:
We have, two identical objects A and B fall from rest from different heights to the ground.
Object B takes twice as long as object A to reach the ground. It is required to find the ratio of the heights from which A and B fell. Let
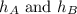 are the height for A and B respectively. So,
are the height for A and B respectively. So,

We have,

So,
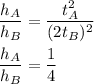
So, the ratio of the heights from which A and B fell is 1:4.