Question

A Florida neighborhood is comprised of a total of 250 houses of which 12% are in foreclosure. A random sample of 91 homes from this neighborhood was selected. The standard error of the proportion is ________.

Answer 1

the standard error of the proportion is 0.0272

Explanation:

We have that if the sample size is greater than 5% of the entire population, a finite population correction factor (fpc) is multiplied with the standard error :

fpc =
`\sqrt{(N -n)/(N -1) }`

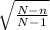
We know that N = 250 n = 91, replacing:

fpc =
`\sqrt{(250 - 91)/(250 -1) }`

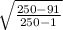
fpc = 0.799

Now, the formula would then be:

SE =
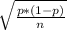`\sqrt{(p * (1 -p))/(n) }`*fpc

Now replacing, knowing that p = 0.12

SE=
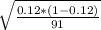`\sqrt{(0.12 * (1 - 0.12))/(91) }`*0.799

SE = 0.0272

So the standard error of the proportion is 0.0272