Answer:
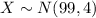
Where
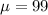 and
and

We want to find the Annie's score takign in count that the score is 3 deviations below the mean, so then we can find the value with this formula:
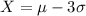
And replacing we got:
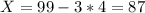
So then the Annie's score would be 87
Explanation:
Let X the random variable that represent the test scores of a population, and for this case we know the distribution for X is given by:
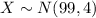
Where
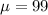 and
and

We want to find the Annie's score takign in count that the score is 3 deviations below the mean, so then we can find the value with this formula:
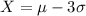
And replacing we got:
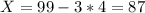
So then the Annie's score would be 87