Question

Among 6 electrical components exactly one is known not to function properly. If 2 components are randomly selected, find the probability that all selected components function properly?

Answer 1

66.67% probability that all selected components function properly

Explanation:

A probability is the number of desired outcomes divided by the number of total outcomes.

The order in which the components are chosen is not important. So we use the combinations formula to solve this question.

Combinations formula:

`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

In this question:

Desired outcomes:

2 components which function properly, from a set of 5. So

`D = C_(5,2) = (5!)/(2!(5-2)!) = 10`

Total outcomes:

2 components, from a set of 6. So

`T = C_(6,2) = (6!)/(2!(6-2)!) = 15`

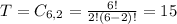
Probability:

`p = (D)/(T) = (10)/(15) = 0.6667`

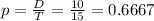
66.67% probability that all selected components function properly