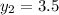Question

The sum of two numbers is 9.9, and the sum of the squares of the numbers is 53.21. What are the numbers?

Answer 1

There are two possibilities:

`x_(1) = 3.5` and
`y_(1) = 6.4`

`x_(2) = 6.4` and
`y_(2) = 3.5`

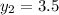
Explanation:

Mathematically speaking, the statement is equivalent to this 2-variable non-linear system:

`x + y = 9.9`

`x^(2) + y^(2) = 53.21`

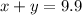
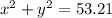
First,
`x` is cleared in the first equation:

`x = 9.9 - y`

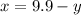
Now, the variable is substituted in the second one:

`(9.9-y)^(2) + y^(2) = 53.21`

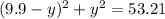
And some algebra is done in order to simplify the expression:

`98.01-19.8\cdot y +2\cdot y^(2) = 53.21`

`2\cdot y^(2) -19.8\cdot y +44.8 = 0`

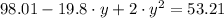
Roots are found by means of the General Equation for Second-Order Polynomials:

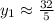`y_(1) \approx (32)/(5)` and
`y_(2) \approx (7)/(2)`

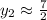
There are two different values for
`x`:

`y = y_(1)`

`x_(1) = 9.9-6.4`

`x_(1) = 3.5`

`y = y_(2)`

`x_(2) = 9.9 - 3.5`

`x_(2) = 6.4`

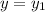
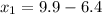
There are two possibilities:

`x_(1) = 3.5` and
`y_(1) = 6.4`

`x_(2) = 6.4` and
`y_(2) = 3.5`