Question

It is known that 25% of inhabitants of a community favour a political party A.

A random sample of 20 inhabitants was selected from the community and each person was asked he/she will vote for party A in an impending election. This follows a Binomial distribution, what is the probability that:
(i) exactly two persons will vote for party A?
(ii) at least three persons will vote for party A?
(iii) fewer than two persons will vote for party A?

Answer 1

i)
`P(X=2)=(20C2)(0.25)^2 (1-0.25)^(20-2)=0.0669`

ii)
`P(X=0)=(20C0)(0.25)^0 (1-0.25)^(20-0)=0.00317`

`P(X=1)=(20C1)(0.25)^1 (1-0.25)^(20-1)=0.0211`

`P(X=2)=(20C2)(0.25)^2 (1-0.25)^(20-2)=0.0669`

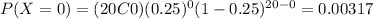
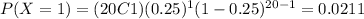
And replacing we got:

`P(X \geq 3) = 1- [0.00317+0.0211+0.0669]= 0.90883`

iii)
`P(X <2)= 0.00317+ 0.0211= 0.02427`

Explanation:

Let X the random variable of interest "number of inhabitants of a community favour a political party', on this case we now that:

`X \sim Binom(n=20, p=0.25)`

The probability mass function for the Binomial distribution is given as:

`P(X)=(nCx)(p)^x (1-p)^(n-x)`

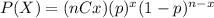
Where (nCx) means combinatory and it's given by this formula:

`nCx=(n!)/((n-x)! x!)`

Part i

We want this probability:

`P(X=2)=(20C2)(0.25)^2 (1-0.25)^(20-2)=0.0669`

Part ii

We want this probability:

`P(X\geq 3)`

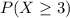
And we can use the complement rule and we have:

`P(X\geq 3) = 1-P(X<3)= 1-P(X \leq 2) =1- [P(X=0) +P(X=1) +P(X=2)]`

And if we find the individual probabilites we got:

`P(X=0)=(20C0)(0.25)^0 (1-0.25)^(20-0)=0.00317`

`P(X=1)=(20C1)(0.25)^1 (1-0.25)^(20-1)=0.0211`

`P(X=2)=(20C2)(0.25)^2 (1-0.25)^(20-2)=0.0669`

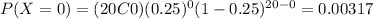
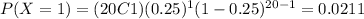
And replacing we got:

`P(X \geq 3) = 1- [0.00317+0.0211+0.0669]= 0.90883`

Part iii

We want this probability:

`P(X <2)= P(X=0) +P(X=1)`

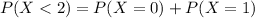
And replacing we got:

`P(X <2)= 0.00317+ 0.0211= 0.02427`