Question

A cellular phone network uses towers to transit calls. Each tower transmits a circular area. on a grid of a city, the equations given represent the transmission boundaries of the towers. Tell which towers, is any, transmit to a phone located at M(3.5, 4.5).

Tower A: x^2 + y^2 = 9

Tower B: (x - 5)^2 + (y - 3)^2 = 6.25

Tower C: (x - 2)^2 + (y - 5)^2 = 4

Answer 1

Towers B and C transmit to the phone.

Explanation:

First, let see the location of the center for each tower:

Tower A

`A (x,y) = (0,0)`

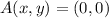
Tower B

`B (x,y) = (5,3)`

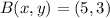
Tower C

`C(x,y) = (2,5)`

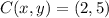
Now, the distance between the location of the phone and any of the towers by means of the Pythagorean equation. The phone is under the influence of a tower only if distance is less than transmission boundaries. Then:

Tower A

`d_(A) = \sqrt{(3.5-0)^(2)+(4.5-0)^(2)}`

`d_(A) \approx 5.701`

`d_(A) > 3`

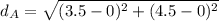
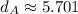
Tower B

`d_(B) = \sqrt{(3.5-5)^(2)+(4.5-3)^(2)}`

`d_(B) \approx 2.121`

`d_(B) < 2.5`

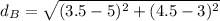
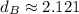
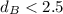
Tower C

`d_(C) = \sqrt{(3.5-2)^(2)+(4.5-5)^(2)}`

`d_(C) \approx 1.581`

`d_(C) < 2`

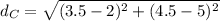
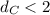
Towers B and C transmit to the phone.

Answer 2

B

Explanation:

Notice that

`(3.5-5)^2 + (4.5-3)^2 = 4.5`

Since 4.5 is less than 6.25, tower B transmits to that phone