Answer:
Slope-intercept form:
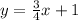
General form:

Explanation:
Firstly, to get the slope intercept form, we need to find the slope and the y-intercept. To find the slope, we can use the slope formula and calculate the "rise over run" for these two points. The coordinate of the first point is (0, 1) , and the coordinate of the second point is (4, 4). If x1 = 0, x2 = 4, y1 = 1, and y2 = 4, we can plug those values into the slope formula that I mentioned earlier, which is
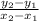 . So, after we plug in those values we get in the two points, we can see that
. So, after we plug in those values we get in the two points, we can see that
 , so the slope is
, so the slope is
 . The x moves right 4 times and the y goes up 3 times. Finally, the y-intercept value is 1 because when x = 0, y is 1, and the point we used for the slope formula (0, 1) proves that. For the slope-intercept form,
. The x moves right 4 times and the y goes up 3 times. Finally, the y-intercept value is 1 because when x = 0, y is 1, and the point we used for the slope formula (0, 1) proves that. For the slope-intercept form,
 , where m is the slope and b is the y-intercept, m is
, where m is the slope and b is the y-intercept, m is
 and b is 1. So, our final answer for the slope-intercept form is
and b is 1. So, our final answer for the slope-intercept form is
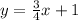 . Now we can find the general form. To do this, we must get one side of the equation to equal 0. Using our slope-intercept equation,
. Now we can find the general form. To do this, we must get one side of the equation to equal 0. Using our slope-intercept equation,
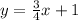 , we can subtract y from both sides to get 0 on the left, like this:
, we can subtract y from both sides to get 0 on the left, like this:
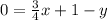 . Then, we can rearrange the variables to general form:
. Then, we can rearrange the variables to general form:
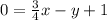 . That is our general form answer. To recap, to find the slope, find the coordinates of the two points, and then plug in those points into the slope formula to find the steepness of the line. Then, add the y intercept that we can see on the graph. For general form, subtract y from both sides and rearrange the equation. Hope this helps!
. That is our general form answer. To recap, to find the slope, find the coordinates of the two points, and then plug in those points into the slope formula to find the steepness of the line. Then, add the y intercept that we can see on the graph. For general form, subtract y from both sides and rearrange the equation. Hope this helps!