Question

An object in the shape of a rectangular prism has a length of 5 inches, a width of 3 inches, and a height of 2 inches. The object's density is 8.3 grams per cubic centimeter. Find the mass of the object to the nearest gram.

Answer 1

`4,080grams`

Explanation:

the formula for density is:

`d=(m)/(V)`

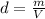
where d is density, m is mass, and V is volume.

solving for m in the last equation:

`m=d*V`

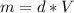
so if we find the volume and multiply it by the density
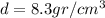`d=8.3gr/cm^3` we will get the mass of the object.

The volume of a rectangular prism is given by:

`V=l*w*h`

where
`l` is length,
`w` is width and
`h` is height:

`l=5in\\w=3in\\h=2in`

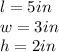
we substitute to find the volume:

`V=5in*3in*2in\\V=30in^3`

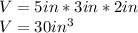
since the density has units of
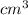`cm^3` we need the volume also in units of
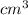`cm^3` , so we make the conversion using:

`1in^3=16.3871cm^3`

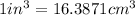
multiplying by 30:

`30in^3=491.612cm^3`

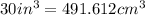
and now we find the mass with the equation:

`m=d*V`

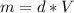
substituting d and V:

`m=(8.3gr/cm^3)(491.612cm^2)\\m=4,080.36gr`

the nearest gram is:
`4,080grams`