Question

Sarah Wiggum would like to make a single investment and have ​$1.7 million at the time of her retirement in 34 years. She has found a mutual fund that will earn 7 percent annually. How much will Sarah have to invest​ today

Answer 1

Sarah has to invest $502,958.58 today.

Explanation:

This is a simple interest problem.

The simple interest formula is given by:

`E = P*I*t`

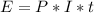
In which E is the amount of interest earned, P is the principal(the initial amount of money), I is the interest rate(yearly, as a decimal) and t is the time.

After t years, the total amount of money is:

`T = E + P`

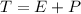
In this question:

`t = 35, I = 0.07, T = 1700000`

She has to invest P today.

`T = E + P`

`1700000 = E + P`

`E = 1700000 - P`

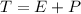
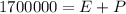
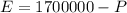
So

`E = P*I*t`

`1700000 - P = P*0.07*34`

`3.38P = 1700000`

`P = (1700000)/(3.38)`

`P = 502958.58`

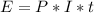
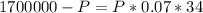
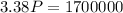
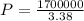
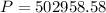
Sarah has to invest $502,958.58 today.