Answer:
a)
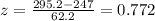
And using the normal distribution table or excel we got:

b)

And we can use the normal standard table or excel in order to find the probability and we got:

Explanation:
For this case we know that the random variable of interest is normally distributed with the following parameters:

Part a
We want to find this probability:

And we can use the z score formula given by:

Replacing we got:
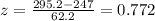
And using the normal distribution table or excel we got:

Part b
We select a random sample of size n = 16 and we try to find this probability:
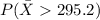
And we can use the z score formula given by:
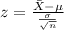
And replacing we got:

And we can use the normal standard table or excel in order to find the probability and we got:
