Answer:
Total weight of blades = ~134gm
Explanation:
Radius of circle,
 = 4 inches
= 4 inches
Central angle of sector =

For finding the weight of blades, we need to find the area of blades.
Area of circle, A =

We know that circle has a central angle of
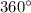 .
.
We can use unitary method to find the area of sector with angle
 .
.
Area of
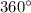 central angle =
central angle =

Area of
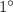 central angle =
central angle =

Area of
 central angle =
central angle =
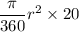
Putting value of r = 4 inches
Area of blade:
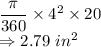
We have 3 blades here, so area of 3 blades =
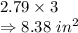
Weight of steel = 16 grams per square inch
Total weight of all 3 blades = 16
 8.38 = ~ 134gm
8.38 = ~ 134gm