Question

Which term of the AP 2,8,14,20..... Will be 210 more than it's 60th term?

Answer 1

95th term

Explanation:

the nth term of an AP is

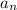`a_(n)` = a₁ + (n - 1)d

where a₁ is the first term and d the common difference

here a₁ = 2 and d = a₂ - a₁ = 8 - 2 = 6 , then

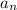`a_(n)` = 2 + 6(n - 1) = 2 + 6n - 6 = 6n - 4

the 60th term is then

a₆₀ = 6(60) - 4 = 360 - 4 = 356

so 210 more than a₆₀ = 356 + 210 = 566

now equate
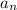`a_(n)` to 566 and solve for n

6n - 4 = 566 ( add 4 to both sides )

6n = 570 ( divide both sides by 6 )

n = 95

Answer 2

95th term.

Explanation:

The sequence of 2, 8, 14, 20 . . . can be predicted by adding 6 to each successive term. This can be written as 6n+2, where n starts at 0 for the first term.

n Value (6n+2)

0 2

1 8

2 14

3 20

4 26

60 362

---

We want to know what term is 210 more than 362. (362+ 210) = 572

We can solve by using the equation in reverse and solving for n:

(6n+2) = 572

6n = 570

n = 95

The 95th term (572) is greater than the 60th term (362) by 210.