Question

A right circular solid cone of base radius 42cm and height 84cm has the same volume as a solid cylinder of height 112cm find the radius of the cylinder

Answer 1

21 cm

Explanation:

Volume of cone is given by
`1/3(\pi r^2h)`

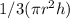
volume of cylinder is given by
`\pi r^2h`

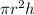
where r is the radius of base and h is the height

_________________________________

For cone

radius = 42cm

height = 84

volume =
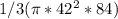`1/3(\pi *42^2*84)` (1)

________________________________

For cone

radius = to find let it be r

height =112

volume =
`\pi *r^2*112` (2)

_______________________________________

since , volume of both solid are same

thus, equation 1 = equation 2

`1/3(\pi *42^2*84) = \pi *r^2*112\\=> r^2 = 1/3((\pi *42^2*84) / 112*\pi \\=> r^2 = 1/3(42*42*84/112) = 441\\=> r = √(441) \\=> r = 21`

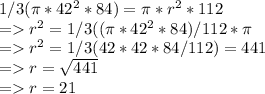
Thus, radius of cylinder is 21 cm