Answer:
Relation between V and c is represented as:
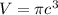
When c is halved, V becomes
 of its initial value.
of its initial value.
Explanation:
Height of cylinder = Radius of cylinder = c
Volume of cylinder = V
As per formula:
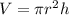
Where
 is the radius of cylinder and
is the radius of cylinder and
 is the height of cylinder
is the height of cylinder
Putting
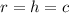
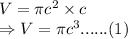
The values of c is halved:
Using equation (1), New volume:

By equation (1), putting
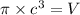
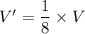
So, when c is halved, V becomes
 of its initial value.
of its initial value.