Answer:
5 boxes of diaries
6 boxes of pencils
10 boxes of rulers
Explanation:
In order to find the smallest number of units that would be possible to buy, find the least common multiple between the number of units in each box:
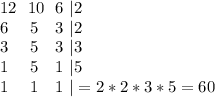
The number of boxes required for each item to get 60 units is:
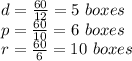
the smallest number of boxes of each item he could buy is:
5 boxes of diaries
6 boxes of pencils
10 boxes of rulers