Answer:
x = 160 ft
Explanation:
The distance traveled by the car between the time the brakes are applied and the time the car comes to a stop is given by:
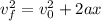
Where:
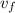 : is the final speed of the car = 0 (since it stops)
: is the final speed of the car = 0 (since it stops)
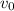 : is the initial speed of the car = 80 ft/s
: is the initial speed of the car = 80 ft/s
a: is the acceleration of the car = -20 ft/s²
x: is the distance recorred by the car
The distance traveled by the car between the time the brakes are applied and the time the car comes to a stop is:
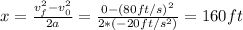
Therefore, the car travels 160 ft between the time the brakes are applied and the time the car comes to a stop.
I hope it helps you!